Projects:ShapeAnalysisWithOvercompleteWavelets
Back to NA-MIC_Collaborations, MIT Algorithms
In this work, we extend the Euclidean wavelets on the sphere. The resulting over-complete spherical wavelets are invariant to rotation of the parameterization of the original spherical image. We apply the over-complete spherical wavelet to cortical folding development and show significantly consistent results as well as improved sensitivity compared with the previous method of using bi-orthogonal spherical wavelet. In particular, we are able to detect developmental asymmetry in the left and right hemispheres.
Description
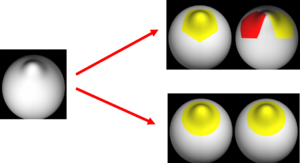
Bi-orthogonal spherical wavelets have been shown to be powerful tools in the segmentation and shape analysis of 2D closed surfaces [1,2], but unfortunately they suffer from aliasing problems and are therefore not invariant under rotations of the underlying surface parameterization. See the toy example in the figure below.
Instead, we propose to use the over-complete spherical wavelets. These over-complete spherical wavelets are based on filter bank theory, directly extending the ideas of Euclidean steerable pyramid to the sphere. We demonstrate the theoretical advantage of over-complete wavelets over bi-orthogonal wavelets. We also show that over-complete spherical wavelets allow us to build more stable cortical folding development models, and detect a wider array of regions of folding development in a newborn dataset.
As seen in the figures above, we see that the bi-orthogonal wavelets analysis of cortical folding is
[1] P. Yu, P. E. Grant, Y. Qi, X. Han, et al., "Cortical surface shape
analysis based on spherical wavelets," IEEE Transaction on Medical
Imaging, vol. 26, pp. 582-97, 2007.
[2] D. Nain, S. Haker, A. Bobick, and A. Tannenbaum, "Multiscale 3-d shape representation and segmentation using spherical wavelets," IEEE Transaction on Medical Imaging, vol. 26, pp. 598-618, 2007.
Key Investigator
MIT: B.T. Thomas Yeo, Peng Yu, Wanmei Ou, Ellent Grant, Bruce Fischl, Polina Golland
Publication
B.T.T. Yeo, W. Ou, P. Golland. On the Construction of Invertible Filter Banks on the 2-Sphere. Yeo, Ou and Golland. Accepted to the IEEE Transactions on Image Processing [In Press]
P. Yu, B.T.T. Yeo, P.E. Grant, B. Fischl, P. Golland. Cortical Folding Development Study based on Over-Complete Spherical Wavelets. In Proceedings of MMBIA: IEEE Computer Society Workshop on Mathematical Methods in Biomedical Image Analysis, 2007.